Second variation formula for minimal surfaces

Posted on August 25, 2009 by Danny Calegari

If $f$ is a smooth function on a manifold $M$ , and $p$ is a critical point of $f$ , recall that the Hessian $H_pf$ is the quadratic form $\nabla df$ on $T_pM$ (in local co-ordinates, the coefficients of the Hessian are the second partial derivatives of $f$ at $p$ ). Since $H_pf$ is symmetric, it has a well-defined index, which is the dimension of the subspace of maximal dimension on which $H_pf$ is negative definite. The Hessian does not depend on a choice of metric. One way to see this is to give an alternate definition $H_pf(X(p),Y(p)) = X(Yf)(p)$ where $X$ and $Y$ are any two vector fields with given values $X(p)$ and $Y(p)$ in $T_pM$ . To see that this does not depend on the choice of $X,Y$ , observe

$X(Yf)(p) - Y(Xf)(p) = [X,Y]f(p) = df([X,Y])_p = 0$

because of the hypothesis that $df$ vanishes at $p$ . This calculation shows that the formula is symmetric in $X$ and $Y$ . Furthermore, since $X(Yf)(p)$ only depends on the value of $X$ at $p$ , the symmetry shows that the result only depends on $X(p)$ and $Y(p)$ as claimed. A critical point is nondegenerate if $H_pf$ is nondegenerate as a quadratic form.

In Morse theory, one uses a nondegenerate smooth function $f$ (i.e. one with isolated nondegenerate critical points), also called a Morse function, to understand the topology of $M$ : the manifold $M$ has a (smooth) handle decomposition with one $i$ -handle for each critical point of $f$ of index $i$ . In particular, nontrivial homology of $M$ forces any such function $f$ to have critical points (and one can estimate their number of each index from the homology of $M$ ). Morse in fact applied his construction not to finite dimensional manifolds, but to the infinite dimensional manifold of smooth loops in some finite dimensional manifold, with arc length as a “Morse” function. Critical “points” of this function are closed geodesics. Any closed manifold has a nontrivial homotopy group in some dimension; this gives rise to nontrivial homology in the loop space. Consequently one obtains the theorem of Lyusternik and Fet:

Theorem: Let $M$ be a closed Riemannian manifold. Then $M$ admits at least one closed geodesic.

In higher dimensions, one can study the space of smooth maps from a fixed manifold $S$ to a Riemannian manifold $M$ equipped with various functionals (which might depend on extra data, such as a metric or conformal structure on $S$ ). One context with many known applications is when $M$ is a Riemannian $3$ -manifold, $S$ is a surface, and one studies the area function on the space of smooth maps from $S$ to $M$ (usually in a fixed homotopy class). Critical points of the area function are called minimal surfaces; the name is in some ways misleading: they are not necessarily even local minima of the area function. That depends on the index of the Hessian of the area function at such a point.

Let $\rho(t)$ be a (compactly supported) $1$ -parameter family of surfaces in a Riemannian $3$ -manifold $M$ , for which $\rho(0)$ is smoothly immersed. For small $t$ the surfaces $\rho(t)$ are transverse to the exponentiated normal bundle of $\rho(0)$ ; hence locally we can assume that $\rho$ takes the form $\rho(t,u,v)$ where $u,v$ are local co-ordinates on $\rho(0)$ , and $\rho(\cdot,u,v)$ is contained in the normal geodesic to $\rho(0)$ through the point $\rho(0,u,v)$ ; we call such a family of surfaces a normal variation of surfaces. For such a variation, one has the following:

Theorem (first variation formula): Let $\rho(t)$ be a normal variation of surfaces, so that $\rho'(0) = f\nu$ where $\nu$ is the unit normal vector field to $\rho(0)$ . Then there is a formula:

$\frac d {dt} \text{area}(\rho(t))|_{t=0} = \int_{\rho(0)} -\langle f\nu,\mu\rangle d\text{area}$

where $\mu$ is the mean curvature vector field along $\rho(0)$ .

Proof: let $T,U,V$ denote the image under $d\rho$ of the vector fields $\partial_t,\partial_u,\partial_v$ . Choose co-ordinates so that $u,v$ are conformal parameters on $\rho(0)$ ; this means that $\langle U,V\rangle = 0$ and $\|U\|=\|V\|$ at $t=0$ .

The infinitesimal area form on $\rho(t)$ is $\sqrt{\|U\|^2\|V\|^2 - \langle U,V \rangle^2} dUdV$ which we abbreviate by $E^{1/2}$ , and write

$\frac d {dt} \text{area}(\rho(t)) = \int_{\rho(t)} \frac {dUdV} {2E^{1/2}} (\|U\|^2\langle V,V\rangle' + \|V\|\langle U,U\rangle' - 2\langle U,V\rangle\langle U,V\rangle')$

Since $V,T$ are the pushforward of coordinate vector fields, they commute; hence $[V,T]=0$ , so $\nabla_T V = \nabla_V T$ and therefore

$\langle V,V\rangle' = 2\langle \nabla_T V,V\rangle = 2\langle \nabla_V T,V\rangle = 2(V\langle T,V\rangle - \langle T,\nabla_V V\rangle)$

and similarly for $\langle U,U\rangle'$ . At $t = 0$ we have $\langle T,V\rangle = 0$ , $\langle U,V\rangle = 0$ and $\|U\|^2 = \|V\|^2 = E^{1/2}$ so the calculation reduces to

$\frac d {dt} \text{area}(\rho(t))|_{t=0} = \int_{\rho(0)} -\langle T,\nabla_U U + \nabla_V V\rangle dUdV$

Now, $T|_{t=0} = f\nu$ , and $\nabla_U U + \nabla_V V = \mu E^{1/2}$ so the conclusion follows. qed.

As a corollary, one deduces that a surface is a critical point for area under all smooth compactly supported variations if and only if the mean curvature $\mu$ vanishes identically; such a surface is called minimal.

The second variation formula follows by a similar (though more involved) calculation. The statement is:

Theorem (second variation formula): Let $\rho(t)$ be a normal variation of surfaces, so that $\rho'(0)=f\nu$ . Suppose $\rho(0)$ is minimal. Then there is a formula:

$\frac {d^2} {dt^2} \text{area}(\rho(t))|_{t=0} = \int_{\rho(0)} -\langle f\nu,L(f)\nu\rangle d\text{area}$

where $L$ is the Jacobi operator (also called the stability operator), given by the formula

$L = \text{Ric}(\nu) + |A|^2 + \Delta_\rho$

where $A$ is the second fundamental form, and $\Delta_\rho = -\nabla^*\nabla$ is the metric Laplacian on $\rho(0)$ .

This formula is frankly a bit fiddly to derive (one derivation, with only a few typos, can be found in my Foliations book; a better derivation can be found in the book of Colding-Minicozzi) but it is easy to deduce some significant consequences directly from this formula. The metric Laplacian on a compact surface is negative self-adjoint (being of the form $-X^*X$ for some operator $X$ ), and $L$ is obtained from it by adding a $0$ th order perturbation, the scalar field $|A|^2 + \text{Ric}(\nu)$ . Consequently the biggest eigenspace for $L$ is $1$ -dimensional, and the eigenvector of largest eigenvalue cannot change sign. Moreover, the spectrum of $L$ is discrete (counted with multiplicity), and therefore the index of $-L$ (thought of as the “Hessian” of the area functional at the critical point $\rho(0)$ ) is finite.

A surface is said to be stable if the index vanishes. Integrating by parts, one obtains the so-called stability inequality for a stable minimal surface $S$ :

$\int_S (\text{Ric}(\nu) + |A|^2)f^2d\text{area} \le \int_S |\nabla f|^2 d\text{area}$

for any reasonable compactly supported function $f$ . If $S$ is closed, we can take $f=1$ . Consequently if the Ricci curvature of $M$ is positive, $M$ admits no stable minimal surfaces at all. In fact, in the case of a surface in a $3$ -manifold, the expression $\text{Ric}(\nu) + |A|^2$ is equal to $R - K + |A|^2/2$ where $K$ is the intrinsic curvature of $S$ , and $R$ is the scalar curvature on $M$ . If $S$ has positive genus, the integral of $-K$ is non-negative, by Gauss-Bonnet. Consequently, one obtains the following theorem of Schoen-Yau:

Corollary (Schoen-Yau): Let $M$ be a Riemannian $3$ -manifold with positive scalar curvature. Then $M$ admits no immersed stable minimal surfaces at all.

On the other hand, one knows that every $\pi_1$ -injective map $S \to M$ to a $3$ -manifold is homotopic to a stable minimal surface. Consequently one deduces that when $M$ is a $3$ -manifold with positive scalar curvature, then $\pi_1(M)$ does not contain a surface subgroup. In fact, the hypothesis that $S \to M$ be $\pi_1$ -injective is excessive: if $S \to M$ is merely incompressible, meaning that no essential simple loop in $S$ has a null-homotopic image in $M$ , then the map is homotopic to a stable minimal surface. The simple loop conjecture says that a map $S \to M$ from a $2$ -sided surface to a $3$ -manifold is incompressible in this sense if and only if it is $\pi_1$ -injective; but this conjecture is not yet known.

Update 8/26: It is probably worth making a few more remarks about the stability operator.

The first remark is that the three terms $\text{Ric}(\nu)$ , $|A|^2$ and $\Delta$ in $L$ have natural geometric interpretations, which give a “heuristic” justification for the second variation formula, which if nothing else, gives a handy way to remember the terms. We describe the meaning of these terms, one by one.

Suppose $f \equiv 1$ , i.e. consider a variation by flowing points at unit speed in the direction of the normals. In directions in which the surface curves “up”, the normal flow is focussing; in directions in which it curves “down”, the normal flow is expanding. The net first order effect is given by $\langle \nu,\mu\rangle$ , the mean curvature in the direction of the flow. For a minimal surface, $\mu = 0$ , and only the second order effect remains, which is $|A|^2$ (remember that $A$ is the second fundamental form, which measures the infinitesimal deviation of $S$ from flatness in $M$ ; the mean curvature is the trace of $A$ , which is first order. The norm $|A|^2$ is second order).
There is also an effect coming from the ambient geometry of $M$ . The second order rate at which a parallel family of normals $\nu$ along a geodesic $\gamma$ diverge is $\langle R(\gamma',\nu)\gamma',\nu\rangle$ where $R$ is the curvature operator. Taking the average over all geodesics $\gamma$ tangent to $S$ at a point gives the Ricci curvature in the direction of $\nu$ , i.e. $\text{Ric}(\nu)$ . This is the infinitesimal expansion of area of a geodesic plane under the normal flow, and has second order. The interactions between these terms have higher order, so the net contribution when $f \equiv 1$ is $\text{Ric}(\nu) + |A|^2$ .
Finally, there is the contribution coming from $f$ itself. Imagine that $S$ is a flat plane in Euclidean space, and let $S_\epsilon$ be the graph of $\epsilon f$ . The infinitesimal area element on $S_\epsilon$ is $\sqrt{1+\epsilon^2 |\nabla f|^2} \sim 1+\epsilon^2/2 |\nabla f|^2$ . If $f$ has compact support, then differentiating twice by $\epsilon$ , and integrating by parts, one sees that the (leading) second order term is $\Delta f$ . When $S$ is not totally geodesic, and the ambient manifold is not Euclidean space, there is an interaction which has higher order; the leading terms add, and one is left with $L = \text{Ric}(\nu) + |A|^2 + \Delta$ .

The second remark to make is that if the support of a variation $f$ is sufficiently small, then necessarily $|\nabla f|$ will be large compared to $f$ , and therefore $-L$ will be positive definite. In other words all variations of a (fixed) minimal surface with sufficiently small support are area increasing — i.e. a minimal surface is locally area minimizing (this is local in the surface itself, not in the “space of all surfaces”). This is a generalization of the important fact that a geodesic in a Riemannian manifold is locally length minimizing (though typically not globally length minimizing).

One final remark is that when $|A|^2$ is big enough at some point $p \in S$ , and when the injectivity radius of $S$ at $p$ is big enough (depending on bounds on $\text{Ric}(\nu)$ in some neighborhood of $p$ ), one can find a variation with support concentrated near $p$ that violates the stability inequality. Contrapositively, as observed by Schoen, knowing that a minimal surface in a $3$ -manifold $M$ is stable gives one a priori control on the size of $|A|^2$ , depending only on the Ricci curvature of $M$ , and the injectivity radius of the surface at the point. Since stability is preserved under passing to covers (for $2$ -sided surfaces, by the fact that the largest eigenvalue of $L$ can’t change sign!) one only needs a lower bound on the distance from $p$ to $\partial S$ . In particular, if $S$ is a closed stable minimal surface, there is an a priori pointwise bound on $|A|^2$ . This fact has many important topological applications in $3$ -manifold topology. On the other hand, when $S$ has boundary, the curvature can be arbitrarily large. The following example is due to Thurston (also see here for a discussion):

Example (Thurston): Let $\Delta$ be an ideal simplex in $\mathbb{H}^3$ with ideal simplex parameter imaginary and very large. The four vertices of $\Delta$ come in two pairs which are very close together (as seen from the center of gravity of the simplex); let $P$ be an ideal quadrilateral whose edges join a point in one pair to a point in the other. The simplex $\Delta$ is bisected by a “square” of arbitrarily small area; together with four “cusps” (again, of arbitrarily small area) one makes a (topological) disk spanning $P$ with area as small as desired. Isotoping this disk rel. boundary to a least area (and therefore stable) representative can only decrease the area further. By the Gauss-Bonnet formula, the curvature of such a disk must get arbitrarily large (and negative) at some point in the interior.

This entry was posted in 3-manifolds, Surfaces and tagged Hessian, Jacobi operator, mean curvature, minimal surface, Morse theory, Riemannian geometry, second variation formula, simple loop conjecture. Bookmark the permalink.

11 Responses to Second variation formula for minimal surfaces

Anonymous says:

August 27, 2009 at 2:48 am

Is it also possible to define the Hessian by applying the exterior derivative twice, $Hf=d^2\!f? In two-dimensional coordinates the calculation would look like$ latex \displaystyle\begin{align*}d^2\!f &= d\left(\frac{\partial f}{\partial x}dx+\frac{\partial f}{\partial y}dy\right)\\ &= \frac{\partial^2\!f}{\partial x^2}dx^2+\frac{\partial^2\!f}{\partial x\partial y}dxdy+\frac{\partial^2\!f}{\partial y\partial x}dydx+\frac{\partial^2\!f}{\partial y^2}dy^2\\ &= \frac{\partial^2\!f}{\partial x^2}dx^2+2\frac{\partial^2\!f}{\partial x\partial y}dxdy+\frac{\partial^2\!f}{\partial y^2}dy^2\end{align*}$

And then observing that this is coordinate independent at critical points.

Reply
Anonymous says:

August 27, 2009 at 2:50 am

Is it also possible to define the Hessian by applying the exterior derivative twice, $Hf=d^2\!f$ ? In two-dimensional coordinates the calculation would look like

$\displaystyle\begin{matrix}d^2\!f &= d\left(\frac{\partial f}{\partial x}dx+\frac{\partial f}{\partial y}dy\right)\\ &= \frac{\partial^2\!f}{\partial x^2}dx^2+\frac{\partial^2\!f}{\partial x\partial y}dxdy+\frac{\partial^2\!f}{\partial y\partial x}dydx+\frac{\partial^2\!f}{\partial y^2}dy^2\\ &= \frac{\partial^2\!f}{\partial x^2}dx^2+2\frac{\partial^2\!f}{\partial x\partial y}dxdy+\frac{\partial^2\!f}{\partial y^2}dy^2\end{matrix}$

And then observing that this is coordinate independent at critical points.

Reply
Danny Calegari says:

August 27, 2009 at 7:29 am

Dear anonymous – not quite sure what you mean: applying the exterior derivative twice to anything gives zero (which is certainly coordinate independent :).

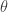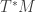In general, to differentiate a 1-form, you need a connection $\nabla$ on the cotangent bundle. If $\theta$ is a 1-form, then $\nabla \theta$ is a section of the second tensor power of $T^*M$ , but one cannot generally say any more than that. If a connection is torsion free (i.e. if the induced connection on $TM$ satisfies $\nabla_X Y - \nabla_Y X - [X,Y]=0$ ) then the antisymmetric part of $\nabla \theta$ is equal to $d\theta$ ; hence $\nabla df$ is always symmetric (but depends on a choice of torsion-free connection). The point is that at a critical point of $f$ , the expression $\nabla df$ does not depend on a choice of connection.

Incidentally, I don’t know what happened to your latex code; are there any readers familiar with latex in wordpress who can say what to fix?

Reply
- Terence Tao says:
  
  August 28, 2009 at 12:26 am
  
  WordPress LaTeX doesn’t support environments such as align*. I suppose something like a \begin{pmatrix} \end{pmatrix} might work instead.
  
  The first variation formula is essential in the Colding-Minicozzi argument showing finite time extinction for Ricci flow, see e.g.
  
  285G, Lecture 4: Finite time extinction of the second homotopy group
  
  The second variation formula isn’t used though, I guess because Ricci flow is first order in time rather than second order. One could speculate though that it could play a role in general relativity, which could crudely be viewed as a variant of Ricci flow that is second order in time.
  
  (The second variation formula for geodesics, on the other hand, is used all over the place in Riemannian geometry, including multiple places in the proof of the Poincare conjecture. This is, of course, the 1-dimensional version of the minimal surfaces second variation formula.)
  
  Incidentally, see you soon in Oz!
  
  Reply
  - Danny Calegari says:
    
    August 28, 2009 at 10:04 am
    
    Dear Terry – thanks for the LaTeX fix (I used \begin{matrix} instead of \begin{pmatrix}) and for the comments on the relationship between first/second variation formula and Ricci flow/GR.
    
    I guess this is a manifestation of the idea that Ricci flow is like an “intrinsic” version of mean curvature flow, and the mean curvature is the gradient of the area functional (by the first variation formula). The fixed points for Ricci flow are Einstein manifolds, whereas the fixed points for mean curvature flow are minimal surfaces; thus in GR (where one studies Einstein manifolds – albeit with a Lorentz signature!) the second order terms are the leading ones, and one can imagine a role for the second variation formula.
    
    Best,
    
    Danny
Anonymous says:

September 23, 2009 at 11:29 am

Could you explicit your second variation formula in the case where S is Euclidian (say RxR)?

I’m just an experimental physicist trying to calculate the parametric surface that describes the shape of a water meniscus, so keeping the technical mathematical terminology to a minimum would be apreciated.

Thanks,
Olivier

Reply
- Danny Calegari says:
  
  September 23, 2009 at 5:04 pm
  
  Hi Olivier – if $S$ is a codimension one minimal surface in some Euclidean space, the Ricci term goes away, and the stability operator reduces to $|A|^2 + \Delta$ . Here $|A|^2$ is just the sum of the squares of the principal curvatures, i.e. $\kappa_1^2 + \kappa_2^2 + \cdots$ and $\Delta$ is the metric Laplacian on $S$ , acting on functions on $S$ , thought of as measuring the size of a (normal) variation. So for example if $S$ is very close to being totally flat, the first term is almost zero, and the only relevant term is $\Delta$ . In other words, the “eigenvariations” of a totally flat (two-dimensional) membrane with fixed boundary are the eigenfunctions of the Laplacian in the domain. For an almost flat surface, thought of as a graph over some domain, the metric Laplacian on the surface will be well-approximated by the ordinary Laplacian in the domain the surface is a graph over; I’m guessing this is the case for a water meniscus?
  
  Best,
  
  Danny
  
  Reply
RoulettGK says:

June 12, 2010 at 3:34 pm

Great idea, thanks for this post!

Reply
Pingback: The Willmore conjecture after Fernando Coda Marques and Andre Neves « Disquisitiones Mathematicae
Pingback: Yau’s conjecture of abundance of minimal hypersurfaces is generically true (in low dimensions) | Disquisitiones Mathematicae
aveliz says:

April 27, 2018 at 6:22 am

Perhaps it is a silly question. I want to understand how the area of a minimal surface changes as one changes the ambient metric. Mpre precisely, is there a way of formulating that change in area in terms of the expression for the second variation using a suitable function $f$ related to the perturbation of the metric $\delta g$.

Best :)

Reply

	tiktok downloader on Bing’s wild involution
	Groups for which qua… on Big mapping class groups and…
	Anonymous on Stiefel-Whitney cycles as…
	Anonymous on Stiefel-Whitney cycles as…
	Israel Socratus Sado… on Bing’s wild involution