I just learned from Jesse Johnson’s blog that Vlad Markovic and Jeremy Kahn have announced a proof of the surface subgroup conjecture, that every complete hyperbolic -manifold
contains a closed
-injective surface. Equivalently,
contains a closed surface subgroup. Apparently, Jeremy made the announcement at an FRG conference in Utah. This answers a long-standing question in
-manifold topology, which is a variation on some problems originally posed by Waldhausen. If one further knew that hyperbolic
-manifold groups were LERF, one would be able to deduce that all hyperbolic
-manifolds are virtually Haken, and (by a recent theorem of Agol), virtually fibered. Dani Wise (and others) have programs to show that hyperbolic
-manifold groups are LERF; if successful, this would therefore resolve some of the most important outstanding problems in
-manifold topology (in fact, I would say: the most important outstanding problems, by a substantial margin).
In fact, the argument appears to work for hyperbolic manifolds of every dimension , and possibly more generally still. Details on the argument of Markovic-Kahn are scarce (Vlad informs me that they expect to have a preprint in a few weeks) but the sketch of the argument presented by Kahn is compelling. Roughly speaking, the argument (as summarized by Ian Agol in a comment at Jesse’s blog) takes the following form:
- Given
, for a sufficiently big constant
, one can find “many” immersed, almost totally-geodesic pairs of pants (i.e. thrice-punctured spheres) with geodesic boundary components (i.e. “cuffs”) of length very close to
. In fact, one can further insist that the complex length of the boundary geodesic is very close to
(i.e. holonomy transport around this geodesic does not rotate the normal bundle very much).
- Conversely, given any geodesic of complex length very close to
, one can find many such pairs of pants that it bounds, and moreover one can find them so that the normal to the geodesic pointing in to the surface is prescribed.
- If one takes a sufficiently big collection of such geodesic pairs of pants, one has enough of them in oppositely-aligned pairs along each boundary component, that they can be matched up (by some version of Hall’s marriage theorem), and furthermore, matched up with a definite prescribed “twist” along the boundary components
- One checks that the resulting (closed) surface is sufficiently close to totally geodesic that the ambient negative curvature certifies it is
-injective
Many aspects of this argument have a lot in common with some previous attempts on the surface subgroup conjecture, including one recent approach by Bowen (note: Bowen’s approach is known to have some fatal difficulties; the “twist” in 3. above specifically addresses some of them). All of these points deserve some comments.
First, where do the pairs of pants come from? If is a totally geodesic pair of pants with boundary components of length close to
, the pants
retract onto a geodesic spine, i.e. an immersed totally geodesic theta graph, whose edges all have length close to
, and which meet at angles very close to
degrees. One can cut this spine up into two pieces, which are obtained by exponentiating the edges of an infinitesimal (almost)-planar tripod for length
.
Given a tripod in some plane in the tangent space at some point of
, one can exponentiate the edges for length
to construct such a half-spine; if
and
are a pair of tripods for which the exponentiated endpoints nearly match up, with almost opposite tangent vectors, then the resulting half-spines can be glued up to make a spine, and thickened to make a pair of pants. One key idea is to use the exponential mixing property of the geodesic flow on a hyperbolic manifold, e.g. as proved by Pollicott. Given some tolerance
, once
is sufficiently large, the mixing result shows that the set of such pairs of tripods for which such a matching occurs have a definite density in the space of all pairs (and in fact, are more and more equidistributed in this space, in probability). In fact, one may even insist that two of the pairs of prongs join up to make some specific closed geodesic of length almost
, and vary the pair of third prongs a very small amount so that they glue up. This takes care of the first two points; this seems quite uncontroversial (exponential mixing comes in, I suspect, to know that one doesn’t need to wiggle the pair of third prongs much, having paired the first two pairs).
The matching (i.e. the gluing up of opposite pant cuffs) apparently is done by some variant of Hall’s marriage theorem. One needs to know (I think) that for any finite set of cuffs to be glued, the set of other cuffs that they could potentially be glued to is at least as big in cardinality. This probably needs some thought, but it is plausibly true: given a cuff, it can be glued to any cuff which is almost oppositely aligned to it, and since there is some tolerance in the angle of gluing — this is where dimension at least is necessary — and moreover, since oriented cuffs are almost equidistributed, one can always find “more” cuffs that are opposite, up to a bit of tolerance, to any given subset of cuffs (of course, more details are necessary here). There is an extra wrinkle to the argument, which is that the gluing must be done with a “twist” of a definite amount, so that cuffs are not glued up in such a way that the perpendicular geodesic arcs joining pairs of cuffs match up.
(Update 8/8: I think there must necessarily be more details to the matching argument, as very loosely described above. There are at least two additional issues that must be dealt with in order to perform a matching: a parity issue (since each pants has an odd number of cuffs) and a homology issue (if the argument relativizes, so that one fixes some collection of cuffs in advance and glues up everything else, one concludes a posteriori that the union of the unglued cuffs is homologically inessential). Probably the parity issue (and more subtle divisibility issues) can be solved by gluing with real-valued weights, then approximating a real solution by a rational solution, and multiplying through to clear denominators. Maybe the homology issue does not arise, if in fact the argument doesn’t relativize.) Both these issues suggest that one does not specify in advance a collection of pants to be glued up, but rather wants to glue up a definite number of pants from some subset.)
This issue of a twist is important for the 4th point, which is perhaps the most delicate. In order to know that the resulting surface is -injective, one must use geometry. A closed (immersed) surface in a hyperbolic manifold which is (locally) very close to being totally geodesic is
-injective. One way to see this is to observe that a geodesic loop in the surface is almost geodesic in the manifold; the ambient negative curvature means that the geodesic can be shrunk (by the negative of the gradient of length in the space of loops) to become geodesic in the ambient manifold; if it is close to being geodesic at the start, it very quickly becomes totally geodesic, without getting much shorter. Any closed geodesic in a hyperbolic manifold is essential.
If one builds a surface by gluing up almost totally geodesic pieces in such a way that there is almost no angle along the gluing, the resulting surface is almost geodesic, and therefore injective. However, one must be very careful to control the geometry of the pieces that are glued, and this is hard to do if the injectivity radius is very small. A geodesic pair of pants has area no matter how long its boundary components are. So if the boundary components have length
, then at the points where they are thinnest, they are only
across. If cuffs are glued where the pants are thinnest, even if the gluing angle is very small, the surfaces themselves might twist through a big angle in a very short time. So one needs to make sure that the thinnest part of one pants are glued up to a thicker part of the next, which is glued to a thicker part of the next . . . and so on. This is the point of introducing the twist before gluing: the twists accumulate, and before one has glued
pieces together, one has entered the thick part of some pants, where the injectivity radius is bounded below by some universal constant.
Anyway, this seems like a really spectacular development, with an excellent chance of working out. Some of the ingredients — e.g. the exponential mixing of the geodesic flow — work just as well in variable negative curvature. In fact, some version of it should work for arbitrary hyperbolic groups (using Mineyev’s flow space). Without knowing more details of the argument, one can’t say how delicate the last part of the argument is, and how far it generalizes (but readers are invited to speculate . . .)

Danny,
Hey, you didn’t tell me you had a blog! Clearly, I need to get one too so I can be one of the cool kids.
Regardless, a nice summary of Kahn and Markovic’s approach — I look forward to reading their preprint.
With regard to LERFness, my (more than second hand) impression is that Wise’s approach requires a Haken manifold to get started. Not that that wouldn’t be major achievement in and of itself — LERF is a hard property to show. For instance even the following absurdly weak statement isn’t known:
Conjecture: Let M be a hyperbolic 3-manifold containing an embedded incompressible surface F. Then M has a finite cover where some component of the preimage of F is non-separating (i.e. is non-trivial in H_2).
The problem is that the two kinds of covers we know about (those coming from congruence quotients and those coming from the fact that (typically) the pro-p completion is non-analytic) aren’t very useful in this context…
Nathan
Hey Nathan – Frank also complained that I didn’t tell anyone about the blog. On the one hand, telling someone about your blog feels a bit self-promoting; on the other hand, why else does one have a blog at all . . . ?
Thanks for filling me in on some details of Wise’s approach to LERF. If you know more details, I’d be very interested to hear them.
Forgive my ignorance, but could you elaborate on the covers coming from non-analytic pro-p completions? Are these (among) the covers in your paper with Frank?
Danny
Well, if you didn’t tell you own brother, I obviously can’t complain that you didn’t tell me. ;-)
The non-analytic pro-p thing I was referring to is Lubotzky’s proof that hyperbolic 3-manifolds do not have the Congruence Subgroup Property. In particular, he showed that if has dimension at least 4, then the pro-p completion of
has dimension at least 4, then the pro-p completion of 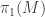 is non-analytic. (Telegraphically the point is that 3-manifold groups always have balanced presentations but those of finite p-groups necessarily have many more relators than generators, i.e. the Golod-Shafarevich inequality) He also showed that any hyperbolic 3-manifold has a congruence cover where
is non-analytic. (Telegraphically the point is that 3-manifold groups always have balanced presentations but those of finite p-groups necessarily have many more relators than generators, i.e. the Golod-Shafarevich inequality) He also showed that any hyperbolic 3-manifold has a congruence cover where  is as large as you want (this is a linear groups thing). Thus virtually the pro-p completion (for fixed p) can always be made non-analytic.
is as large as you want (this is a linear groups thing). Thus virtually the pro-p completion (for fixed p) can always be made non-analytic.
My paper with Frank exploits, it turns out, the non-generic situation: what Boston and Ellenberg noticed is that in our example the pro-3 completion is analytic and thus that taking 3-group covers can’t increase .
.
The reason p-group covers aren’t good for “baby-LERF” is just this. What we need to make non-separating are finite quotients
non-separating are finite quotients  where
where  does not surject. But a set of elements generate a p-group if and only if they generate its abelianization. Thus p-group covers don’t seem to be any more useful than plain abelian covers. (Congruence covers will fail unless
does not surject. But a set of elements generate a p-group if and only if they generate its abelianization. Thus p-group covers don’t seem to be any more useful than plain abelian covers. (Congruence covers will fail unless  is totally geodesic since
is totally geodesic since  is Zariski dense and thus surjects onto almost every congruence quotient by Nori-Weisfeiler Strong Approximation. )
is Zariski dense and thus surjects onto almost every congruence quotient by Nori-Weisfeiler Strong Approximation. )
Thanks Nathan – very informative.
Best,
Danny
This is not really on-topic, but why is Clay muscling in on the Mahler lectures, something whose ultimate purpose, surely, should be a sinecure for expatriate Australian number theorists? (especially those that have cited Mahler’s actual papers.)
I think for this year only, they decided to combine the Mahler lectures with the Clay lectures; so Terry Tao (who certainly qualifies as an expat that has made significant contributions to number theory) is the Clay-Mahler lecturer, whereas Mohammed Abouzaid and I are merely Clay lecturers.
Pingback: Surface subgroups – more details from Jeremy Kahn « Geometry and the imagination
Pingback: Agol’s Virtual Haken Theorem (part 1) « Geometry and the imagination
Pingback: Exciting News on Three Dimensional Manifolds | Combinatorics and more
Pingback: Cube complexes, Reidemeister 3, zonohedra and the missing 8th region « Geometry and the imagination