Agol’s Virtual Haken Theorem (part 2): Agol-Groves-Manning strike back

Today Jason Manning gave a talk on a vital ingredient in the proof of Agol’s theorem, which is a result in geometric group theory. The theorem is a joint project of Agol-Groves-Manning, and generalizes some earlier work they did a few years ago. Jason referred to the main theorem during his talk as the “Goal Theorem” (I guess it was the goal of his lecture), but I’m going to call it the Weak Separation Theorem, since that is a somewhat more descriptive name. The statement of the theorem is as follows.

Weak Separation Theorem (Agol-Groves-Manning): Let G be a hyperbolic group, let H be a subgroup of G which is quasiconvex, and isomorphic to the fundamental group of a virtually special NPC cube complex, and let g be an element of G which is not contained in H. Then there is a surjection $\phi:G \to \bar{G}$ so that

$\bar{G}$ is hyperbolic;
$\phi(H)$ is finite; and
$\phi(g)$ is not contained in $\phi(H)$ .

In the remainder of this post I will try to explain the proof of this theorem, to the extent that I understand it. Basically, this amounts to my summarizing Manning’s talk (or the part of it that I managed to get down in my notes); again, any errors, foolishness, silly blog post titles etc. are due to me.

Recall from my previous post that an NPC (non-positively curved) cube complex is a compact quotient of a CAT(0) cube complex by a group (in this case H) acting properly discontinuously, and that the complex is virtually special if it has a finite (orbi-)cover satisfying the Haglund-Wise conditions (i.e. hyperplanes are embedded, two-sided, and there are no self- or interosculations). The fundamental group of a virtually special cube complex is a linear group (i.e. embeds in $\text{GL}(n,\mathbb{C})$ for some n), and is therefore residually finite; this means that the intersection of all finite index normal subgroups consists only of the identity element. The fact that all finitely generated linear groups are residually finite is known (to topologists, anyway) as Selberg’s Lemma. Roughly, the idea is to consider the ring A of matrix entries in a faithful linear representation, and then the desired finite index subgroups are the kernels of maps to $\text{GL}(n,A/\mathfrak{p})$ for suitable prime ideals $\mathfrak{p}$ in $A$ (see e.g. here for more details). More generally, if G is a group, a subgroup H is said to be separable if for any g not in H there is a homomorphism from G to a finite group so that the image of g is disjoint from the image of H. Groups in which every finitely generated subgroup is separable are said to be LERF; it is a consequence of Agol’s proof of the VHC that every hyperbolic 3-manifold group is LERF, but we are getting ahead of ourselves here.

The Weak Separation Theorem is in the direction of showing that the subgroup H is separable; if the quotient $\bar{G}$ could be taken to be finite, that is exactly what it would show. But finding a quotient where $\phi(H)$ is finite turns out to be good enough for Agol’s purposes.

An important special case of the theorem is when H is almost malnormal. A subgroup H of G is normal if conjugation in G sends H to itself. H is malnormal if $H^g \cap H = \text{id}$ for all $g \in G-H$ , where superscript denotes conjugation, and H is almost malnormal if $H^g \cap H$ is finite for all $g \in G-H$ .

Bowditch showed that if G is hyperbolic and H is quasiconvex and almost malnormal in G, then the pair $(G,\lbrace H \rbrace)$ is relatively hyperbolic. The concept of relative hyperbolicity generalizes the fundamental group of a noncompact complete negatively curved manifold of finite volume; the fundamental group is not (necessarily) a hyperbolic group (although it is in some cases!) but the lack of hyperbolicity is concentrated in the noncompact cusp of the manifold; the fundamental group of the cusp itself might or might not be hyperbolic. It is a parabolic subgroup of the fundamental group, and the pair is relatively hyperbolic. Abstractly, a pair $(G,\mathscr{P})$ is relatively hyperbolic, where $\mathscr{P}$ is a collection of conjugacy classes of subgroups of $G$ , if the space obtained by attaching “horoballs” to the conjugates of the subgroups in $\mathscr{P}$ in (the Cayley graph of) G is hyperbolic (see here for more details). Note that if the subgroups $\mathscr{P}$ are themselves hyperbolic, then $G$ is also hyperbolic (in the absolute sense).

Relatively hyperbolic groups invite relatively hyperbolic Dehn filling, by analogy with Thurston’s hyperbolic Dehn surgery for (noncompact) hyperbolic 3-manifolds with cusps. Suppose $(G,\mathscr{P})$ is relatively hyperbolic, where $\mathscr{P}=\lbrace P_1,\cdots,P_m\rbrace$ . For each i choose some normal subgroup $N_i$ of $P_i$ . The quotient of $G$ by the normal closure (in G) of all the $N_i$ is called a filling of G by the $N_i$ , and is denoted $G(N_1,\cdots,N_m)$ . If each of the $N_i$ is finite index in $P_i$ , we call it a peripherally finite filling. The fundamental theorem of hyperbolic Dehn surgery, due (in this form) originally to Osin, is as follows:

Theorem (Osin): Let F be a finite subset of G, and let $(G,\mathscr{P})$ be relatively hyperbolic. Then there is a finite subset B of G, so that for any filling $\phi:G \to G(N_1,\cdots,N_m)$ for which B does not intersect any of the $N_i$ , one has the following:

$\phi(P_i) = P_i/N_i$ for each i;
the image pair $(\bar{G},\bar{\mathscr{P}})$ in the quotient is relatively hyperbolic
the restriction of $\phi$ to $F$ is injective.
$\phi(F)\cap \phi(P_i) = \phi(F\cap P_i)$ for all i

Actually, the fourth condition is not proved by Osin, but can be deduced with “a bit of work”, according to Jason. Notice that if the filling is peripherally finite, so that all $P_i/N_i$ are finite, then these quotients of the parabolic groups are trivially hyperbolic (since every finite group is hyperbolic), and therefore the quotient group $\bar{G}$ is also hyperbolic.

If H is almost malnormal, Osin’s surgery theorem implies the Weak Separability Theorem, as follows. Since H is almost malnormal and quasiconvex, and G hyperbolic, by Bowditch the pair $(G,\lbrace H \rbrace)$ is relatively hyperbolic. We let F (as in the statement of Osin’s theorem) consist only of the element $g$ , and let $B$ be the finite set of “bad” fillings the theorem guarantees. Since H is assumed to be virtually special, it is residually finite, and therefore contains a finite index normal subgroup N missing B. Taking the quotient of G by the normal closure of N gives a surjection to a group in which the image of H is finite, and disjoint from the image of g, as desired.

What if H is not malnormal? Then one must induct on an invariant called the height, which measures the failure of the group to be almost malnormal. The idea of height was introduced by Gitik-Mitra-Rips-Sageev.

Definition: If H is quasiconvex in a hyperbolic group G, the height of H is the least integer n so that if there are elements $g_1,\cdots,g_n$ so that $H,g_1H,\cdots,g_nH$ are distinct, then the intersection of conjugates $H\cap H^{g_1} \cap \cdots \cap H^{g_n}$ is finite.

H has height 0 if and only if it is finite. It has height 1 if and only if it is almost malnormal and infinite. One can define a complex whose k-simplices are the $(k+1)$ -fold infinite intersections of distinct conjugates of H, and height is then the dimension of this complex plus one. Gitik-Mitra-Rips-Sageev prove that every quasiconvex subgroup of a hyperbolic group has finite height. They also show that for any k there are only finitely many H-conjugacy classes of infinite groups of the form $H\cap H^{g_1}\cap \cdots \cap H^{g_k}$ (this is vacuous for k as big as the height or bigger). The minimal such infinite intersections are not far from being malnormal, and after a suitable modification, will give rise to a suitable relatively hyperbolic family.

Start with $\mathscr{D}''$ , the collection of H-conjugacy classes of minimal infinite intersections of the form $H \cap H^{g_1} \cap \cdots \cap H^{g_k}$ (these are conjugacy classes of subgroups in H). These subgroups are intersections of quasiconvex subgroups, and are easily seen to be quasiconvex themselves. Replace each D in $\mathscr{D}''$ by its commensurator in H (note that each D is finite index in $\text{comm}_H(D)$ ), and then choose one such subgroup per H-conjugacy class. This produces a new collection of conjugacy classes of subgroups $\mathscr{D}$ in H. Observe that the elements of $\mathscr{D}$ are almost malnormal — in fact $\mathscr{D}$ is an almost malnormal collection, meaning that nontrivial conjugates of one subgroup intersect any other subgroup in the collection in a finite set — so that $(H,\mathscr{D})$ is relatively hyperbolic (Bowditch’s criterion for relative hyperbolicity generalizes to almost malnormal collections of quasiconvex subgroups).

Now, replace each D in $\mathscr{D}$ by its commensurator in G; again each D is finite index in $\text{comm}_G(D)$ . This produces a collection $\mathscr{P}'$ of subgroups of G; choose one subgroup for each G-conjugacy class to arrive finally at an almost malnormal collection $\mathscr{P}$ of quasiconvex subgroups of G, and deduce (by Bowditch again) that $(G,\mathscr{P})$ is relatively hyperbolic.

Definition: A filling $G \to G(N_1,\cdots, N_m)$ with each $N_i$ in some $P_i$ in $\mathscr{P}$ as above is an H-filling if whenever $D\cap P_i^g$ is infinite for some $D$ in $\mathscr{D}$ , then $N_i^g$ is contained in D.

An H-filling by definition induces a filling of H, i.e. a quotient $H \to H(N_{i_1}^{g_1},\cdots,N_{i_k}^{g_k})$ . With this terminology, Agol-Groves-Manning prove

Theorem (Agol-Groves-Manning): Let G be hyperbolic, let H be quasiconvex in G of height at least 1, and let $(G,\mathscr{P})$ and $(H,\mathscr{D})$ be as above, and let g be in $G-H$ . Then for all “sufficiently long” peripherally finite H-fillings $\phi: G \to G(N_1,\cdots,N_m)=:\bar{G}$ ,

$\phi(H)$ is isomorphic to the induced filling of H;
$\phi(H)$ is quasiconvex in $\bar{G}$ ;
$\phi(g)$ is not contained in $\phi(H)$ ; and
the height of $\phi(H)$ is strictly less than the height of H.

Let’s not worry too much about what the condition “sufficiently long” means here; suffice it to say that such fillings can be found if H is residually finite.

Now, in the setup of the Weak Separation Theorem, the subgroup H is the fundamental group of a virtually special NPC complex, and is therefore residually finite. So we can apply this filling theorem of AGM to reduce the height of H. But now one is stuck, because the resulting image $\phi(H)$ , while of strictly lower height, might not be residually finite. Here is where Wise’s Malnormal Special Quotient Theorem (alluded to at the end of my previous post) comes in. The statement of the MSQT is as follows:

Malnormal Special Quotient Theorem (Wise): Let H be hyperbolic, let $(H,\mathscr{D})$ be relatively hyperbolic, where the $D_i$ in $\mathscr{D}$ are almost malnormal and quasiconvex. Suppose H is the fundamental group of a virtually special NPC complex. Then there are finite index subgroups $\dot{D}_i$ in the $D_i$ so that if $\phi:H \to H(N_1,\cdots,N_m)$ is any peripherally finite filling with $N_i$ contained in the $\dot{D}_i$ , then $\phi(H)$ is the fundamental group of a virtually special NPC complex.

The MSQT implies that, providing we are careful for our H-filling to kill subgroups contained in the $\dot{D}_i$ , the image of H will be virtually compact special, and the induction can be continued, reducing the height of (the image of) H until the Weak Separation Theorem is proved.

15 Responses to Agol’s Virtual Haken Theorem (part 2): Agol-Groves-Manning strike back

Mayer A. Landau says:

March 27, 2012 at 5:34 pm

You state “it is a consequence of Agol’s proof of the VHC that every 3-manifold group is LERF”. I thought there were some graph manifold fundamental groups that were not LERF. Is that incorrect?

- Danny Calegari says:
  
  March 27, 2012 at 10:32 pm
  
  Sorry – I meant to write “every hyperbolic 3-manifold group is LERF”. I’ve changed it; thanks for the correction.
  
- dfuter says:
  
  April 1, 2012 at 1:52 pm
  
  In the same spirit as Mayer’s question: there are a bunch of hyperbolic 3-manifolds whose fundamental groups are not actually hyperbolic (they are hyperbolic relative to the cusp subgroups). Do you have a sense of whether Ian’s work applies to these cusped manifolds? Are these groups also LERF/large?
  
  - Danny Calegari says:
    
    April 2, 2012 at 12:14 am
    
    Hi Dave – If M is cusped hyperbolic, it can be Dehn filled to be closed hyperbolic. The result then has a finite cover whose fundamental group is large; this induces a finite cover of M whose fundamental group is similarly large.
    
    I think the same should be nearly true for subgroup separability. Let G=pi_1(M), H be a finitely generated relatively quasiconvex subgroup, and g an element in G-H. Providing g is not itself contained in a peripheral subgroup, I believe we can Dehn fill the cusps to get a closed hyperbolic manifold where the image of g is still disjoint from the image of H. Then separate the images in a further finite quotient (it doesn’t matter if H is quasiconvex in the image, but I think we can arrange it anyway). I don’t know if one can separate H and g if they both nontrivially intersect the same peripheral subgroup (for instance, if the intersection of H with a peripheral subgroup has finite index), but I expect this case can be analyzed.
    
    Anyway, I’m just speaking off the cuff here (off the cusp?) so take this with a grain of salt.
  - Jason Manning says:
    
    April 3, 2012 at 6:15 am
    
    Danny’s correct. LERF-ness for closed hyperbolic implies LERF-ness for cusped hyperbolic. You can find details in my paper with Eduardo Martinez-Pedroza, arXiv:0811.4001, Proposition 5.3.
  - dfuter says:
    
    April 3, 2012 at 5:34 pm
    
    Thank you, Danny and Jason!
  - Henry Wilton says:
    
    April 5, 2012 at 7:06 am
    
    I believe that one doesn’t need to appeal to Agol for either largeness or LERF in the cusped case – it already followed from Wise’s work. (Though there is a caveat. See my final paragraph.)
    
    In a previous incarnation of Wise’s preprint, he stated as a theorem that fundamental groups of cusped hyperbolic 3-manifolds are virtually *compact* special. (In some relatively hyperbolic contexts, one only has something called *sparsely* special, which is technically much more tricky.) This was Theorem 17.1 in the first version I saw, and Theorem 16.1 in some subsequent versions. Eric Chesebro, Jason DeBlois and I used Eduardo and Jason’s work to prove that if a cusped hyperbolic 3-manifold is virtually compact special then every geometrically finite subgroup is a virtual retract. In particular, every geometrically finite subgroup is separable (so LERF follows from tameness) and the fundamental group virtually retracts onto any non-abelian, free, geometrically finite subgroup, such as a Schottky subgroup.
    
    However, as mentioned above, there is a caveat. When looking at the latest version of the manuscript linked to from Jason Behrstock’s web page today, I notice that I can no longer find these statements in the current version! This is quite concerning, as for other applications (eg conjugacy separability) one definitely needs to know that that these groups compact special. I will have to find out exactly what Wise currently claims.
  - Henry Wilton says:
    
    April 5, 2012 at 1:40 pm
    
    On further inspection, I have managed to find the relevant theorem again in Wise’s preprint. It’s currently Theorem 14.29, which asserts: ‘Let M be a finite volume cusped hyperbolic 3-manifold. Then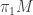 $\pi_1M$ is virtually the fundamental group of a compact special cube complex.’
    
    For reference, I’m referring to the manuscript ‘The structure of groups with a quasiconvex hierarchy’. You can access this on google docs here.
  - Henry Wilton says:
    
    April 19, 2012 at 3:21 am
    
    Two more comments:
    
    1. Cusped hyperbolic 3-manifolds were already known to be large by Cooper–Long–Reid.
    
    2. I don’t think you can deduce virtual fibredness from the closed case via Dehn filling. Unfortunately, one only knows that closed hyperbolic 3-manifold groups are virtually RFRS, and so Dehn filling only gives you that cusped hyperbolic 3-manifolds are residually virtually RFRS. This isn’t prima facie enough to deduce virtual-fibredness.
  - dfuter says:
    
    April 19, 2012 at 2:23 pm
    
    Henry: thanks for all your thoughts here. As for virtual fibering of cusped 3-manifolds, I assume the proper thing here is to credit Wise?
  - Henry Wilton says:
    
    April 20, 2012 at 5:07 am
    
    Dave: exactly.
ianagol says:

March 29, 2012 at 11:50 pm

I think “The Empire strikes back” was actually part V.

- Danny Calegari says:
  
  March 30, 2012 at 8:01 am
  
  Everyone’s a critic . . .
  
Pingback: Exciting News on Three Dimensional Manifolds | Combinatorics and more
Pingback: ICM2014 — Ian Agol plenary lecture | Gowers's Weblog

	tiktok downloader on Bing’s wild involution
	Groups for which qua… on Big mapping class groups and…
	Anonymous on Stiefel-Whitney cycles as…
	Anonymous on Stiefel-Whitney cycles as…
	Israel Socratus Sado… on Bing’s wild involution